SciGen Teacher Dashboard

Unit E4

Making Waves

Surf's Up (and Down, and Up Again)

A Wave with Words

Modeling Mechanical Waves

Ripple Tank

Sound Stations

Waves of Danger

Lesson: Modeling Mechanical Waves

Duration: Approximately 115 minutes

In this activity, students explore the nature of waves using two models: one transverse and the other longitudinal.

LEARNING OBJECTIVES

Students understand the difference between transverse and longitudinal waves.

Teacher Tips

If your students don't remember the terms "perpendicular" and "parallel" from math class, click here for a visual explanation.
For the context-setting opening piece, you might consider looking at a more in-depth lesson about how to demonstrate waves on a spring like a Slinky. Exploratorium physics teacher Paul Doherty's Slinky in Hand activity captures many of the demos you might want to do with the Slinky, as have seismologists in Michigan Tech, but look around online because many teachers have written up this project! (Even Paul Doherty wrote about these Slinky wave models with different details a couple of decades earlier.)

Materials

1–2 long spring toys (like a Slinky)
electronic device (such as a computer, laptop, or tablet) to access animated models

Teacher Tune-ups

Teaching Notes

ACTIVITY OVERVIEW

Start with a Slinky for context (5 minutes)
Explore two wave models (10 minutes)
Act out a wave as a class (10 minutes)
Explore the Water Wave Model (15 minutes)
Discussion: comparing to other models (10 minutes)
Wrap up (5 minutes)

Start with a Slinky for context (5 minutes)

Paraphrase:

Today we are going to learn about the differences between two mechanical waves.

Use one or two Slinky toys to demonstrate two kinds of mechanical waves: transverse and longitudinal. Transmit energy down the Slinky or Slinkys.

Explore two wave models (10 minutes)

Paraphrase:

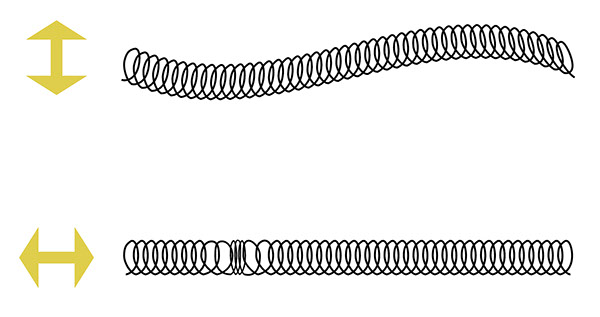
One way to categorize waves is by the way particles in the wave move. These are two type of waves.

With a partner, please play with these two models. Try out all the buttons, and try changing the color of some of the dots by clicking on them.

Using the focus words and other academic language you know, attempt to describe in your lab book what you observe as the same or different when examining these waves.

Use the concepts of parallel and perpendicular to identify Wave Model A and Wave Model B by their scientific names.

Act out a wave as a class (10 minutes)

Paraphrase:

We've all seen waves like the ones below at sports events live or on television.

Is this an example of transverse or a longitudinal wave? Explain your thinking to your partner.

Is there is way to increase and decrease the transverse wave's frequency, wavelength, or amplitude?

Create a wave with low frequency. Solution: students stand and sit slowly.
Create a wave with high frequency. Solution: students stand and sit quickly.

Create a wave with a long wavelength. Solution: students stand and sit slowly.
Create a wave with a short wavelength. Solution: students stand and sit quickly.

What's the relationship between frequency and wavelength?

Possible answer:

As long as the speed of the wave is the same, frequency and wavelength are inversely related: as one gets bigger, the other gets smaller, and vice versa.

Create a wave with medium amplitude. Solution: students stand up and sit down.
Create a wave with high amplitude. Solution: students stand up and raise arms before sitting down.
Create a wave with low amplitude. Solution: students sit up as tall as they can, then return to resting state.

Frequency Challenges

Create a wave with low frequency.
Create a wave with high frequency.

Wavelength Challenges

Create a wave with a long wavelength.
Create a wave with a short wavelength.

Amplitude Challenges

Create a wave with medium amplitude.
Create a wave with high amplitude.
Create a wave with low amplitude

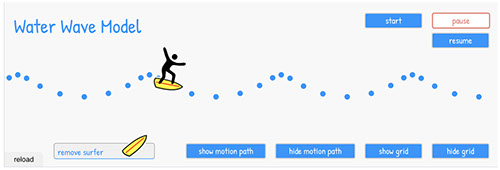
Explore the Water Wave Model (15 minutes)

Paraphrase:

Here is a model of the surface of water waves. Examine the model closely and observe the motion of the dots.

Ask:

Are water waves transverse? Longitudinal? Or maybe something else? Come up with the best description you can.

Ask students:

Use the Water Wave Model to begin constructing a theory about why the beach ball and the surfer interact with the wave so differently.

This is tricky! Good luck! Be ready to present your thinking during a class discussion.

Possible response:

Surfers stay on the front of a wave and angle the surfboard so that they catch the wave's energy to move forward.

A beach ball is very light, so it will mostly follow the motion of the water it is sitting on. Since the same amount of water moves backwards and forwards, the ball does not keep moving in the same direction.

Surfers who are not standing on their boards will move like the beach ball and bob up and down.

Paraphrase:

Sometimes people get the wrong idea about water waves when watching surfers. They think that since a surfer is moving quickly toward the shore, the water must also be moving toward the shore along with surfer.

Look carefully at the model. Is the water moving?

What is happening in the model? (extended Teaching Notes)

Surfers deliberately stay on the front slope of their wave (by swimming and choosing when to stand up), and angle their surfboards so that the rate of toward-the-shore motion matches the rate at which the water underneath them rises due to the wave action of the water. Since the rising water is in the part of its circular path that is moving toward the shore, surfers keep moving the direction they want even though each part of the water is moving back and forth. This strategy explains why surfers go forward but not backward, even though equal amounts of the water they are riding on go both ways (ignoring tides).

Because (in the absence of wind) a beach ball does not have any force acting on it, it stays on the front slope of a wave. The ball is also very light, so in the absence of wind it will tend to follow the motion of the water it is sitting on and thus move in a circular path rather than moving toward the shore. In practice, the movement of a beach ball will be determined by even a gentle wind, since it is very light, has a large area, and will quickly return to the surface even if it is briefly submerged by a breaking wave.

The actual water molecules in ocean waves move in paths that are usually nearly circular. In the shallow water close to shore, however, friction slows the water motion near the bottom so that the water near the surface moves faster, forming a "breaking" wave when the top gets ahead of the water that was supporting it.

What about crashing waves coming onto the shore?

Students may wonder "Why do waves break at the beach?" or ask about the difference between waves in the open ocean and those at the beach. They may note that the water of a crashing wave moves forward, not just up and down, as waves come ashore.

Waves break when the top of the wave is traveling faster than the bottom of the wave. As a wave moves towards a beach, the depth becomes shallower and the bottom of the wave slows down. Waves start to break when the depth of the water is just 30% more than the height of the wave. The top of the wave continues to move forward faster than the bottom of the wave, and the energy of the wave gets pushed up as well. A complicated combination of forces creates the curling lip of a wave.

Water does move a great deal at the shore, onto the beach and back again, but it does not continue moving forward. After crashing on the shore, the water that moved forward with the excess energy of the wave returns to the ocean or lake to come forward with the wave.

This level of understanding is not important for your students to have at this time, but some perceptive students may ask about waves.

Discussion: comparing to other models (10 minutes)

"Water waves are an example of waves that involve a combination of both longitudinal and transverse motions. As a wave travels through the water, the particles travel in clockwise circles. The radius of the circles decreases as the depth into the water increases. The animation at right shows a water wave traveling from left to right in a region where the depth of the water is greater than the wavelength of the waves. I have identified two particles in orange to show that each particle indeed travels in a clockwise circle as the wave passes."—Professor Dan Russell

Ask:

Was your idea about classifying water waves on the right track?

Show the second slide to compare Dan Russell's other models to the animated terms you may have worked with previously in this unit. Encourage your students to focus on random, individual particles (comparing individuals in the group) so that they trust that the illusion of dense “lines” of particles moving across the screen to the right, is in fact the cycling of particles shown in the previous demonstration. Note that the slide includes a label with the term “rarefaction.” If students wonder about this term, challenge them to come up with a definition for it, by comparing it to the areas labeled "compression." Recall that compression is defined as "the state of being squeezed or pressed together." Rarefaction, then, would be something like "the state of being pulled apart."

Ask:

How do Professor Russell's models compare to the other models we've used?

Prompt students to look at the behavior and movement of single particles and then to step back and look at the behavior at a distance.

Professor Russell's water wave model

Professor Russell's longitudinal wave models

Professor Russell's transverse wave model

Writing: Water flow vs. wave motion (5 minutes)

To wrap up, return to the two videos from the beginning of E4.2 Interactive: A Wave with Words.

Both show moving water. Ask students to describe how the two videos differ in writing, using some of the unit's focus words and what they experienced in class today.

Which one shows wave behavior?

Only one of the videos above is a good example of waves.
Which one? Why?

Use the Focus Words in your writing. They can help you make a better argument.

wave • frequency • amplitude • trough • crest • wavelength • compression • medium

BETA Version - Please send comments and corrections to info@serpinstitute.org

This project was supported by Science Sandbox, a Simons Foundation initiative dedicated to engaging everyone with the process of science.

Strategic Education Research Partnership

1100 Connecticut Ave NW Suite 1310 • Washington, DC 20036 • (202) 223-8555

info@serpinstitute.org